Types of assumptions in Lorenz curve
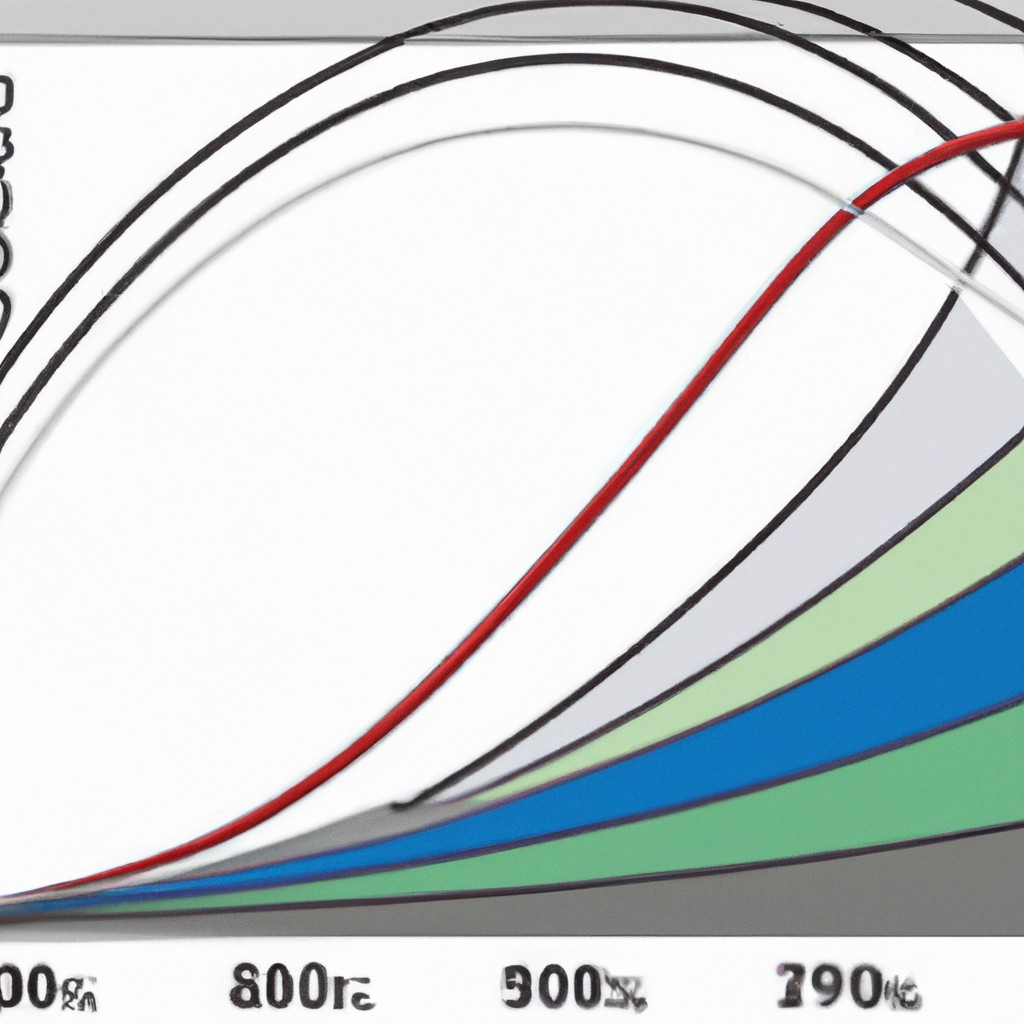
When analyzing the Lorenz curve, it is important to consider various assumptions. One key assumption is the linearity between income percentile and cumulative income proportion. Another assumption involves the inequality measure used to calculate the Gini coefficient. Additionally, it is assumed that income distribution is stable over time for accurate comparisons. Finally, there is the assumption of income data accuracy and representativeness to ensure reliable results. These assumptions guide the interpretation of the Lorenz curve and provide a framework for understanding income inequality dynamics. Understanding these assumptions is crucial for meaningful analysis and policy formulation related to income distribution.
Read more
Assumptions
Assumptions can lead us astray, coloring our perceptions and limiting our understanding of others. Sometimes our assumptions are based on incomplete information or biased perspectives, clouding our judgment and hindering meaningful connections. It's essential to recognize when we are making assumptions and challenge them with open-mindedness and curiosity. By questioning our assumptions and seeking to truly understand different viewpoints, we can foster empathy and build stronger relationships. Letting go of assumptions can free us from preconceived notions and allow for deeper, more authentic interactions with others. Embracing uncertainty can lead to growth and richer, more fulfilling experiences.
Read more
Assumptions of the Atkinson index
The Atkinson index assumes unequal incomes distribution. It measures the impact of income inequality. A lower Atkinson score indicates less inequality. It accounts for social welfare loss from inequality. This index considers individual utility functions. It assumes a diminishing marginal utility of income. The Atkinson index reflects fairness perceptions. It has been criticized for disregarding poverty. Despite limitations, it's used in income distribution analysis. Policymakers rely on it for decision-making. The Atkinson index shapes social policies. Understanding its assumptions is crucial. It influences strategies to address inequality. Public debate often centers on its implications. The Atkinson index is a vital tool.
Read more
Assumptions underlying the Atkinson Index
The Atkinson Index evaluates income inequality while considering the distribution's shape. It assumes individuals care about inequality. It assumes diminishing marginal utility of income. It assumes equal weights on each person. These assumptions drive its formulation and interpretations. Critics debate these foundational assumptions. They question their real-world accuracy and applicability. Despite criticisms, the Atkinson Index remains widely used. Policymakers and researchers find it valuable for understanding inequality trends. Its simplicity and focus on distributional shape make it accessible. By highlighting inequality dynamics, it sheds light on social and economic disparities. The Atkinson Index is a powerful tool for analyzing income distribution and guiding policy decisions.
Read more
Assumptions and limitations of Lorenz curve
The Lorenz curve is a powerful tool used in economics to visualize income distribution. However, it comes with assumptions and limitations. First, it assumes a closed economy, ignoring international income disparities. Second, it assumes that income is the only measure of well-being, disregarding other factors like education and healthcare. Third, it assumes that the distribution of income is static over time, neglecting any changes that might occur. Additionally, the Lorenz curve cannot capture the complex dynamics of an economy, such as the effects of economic policies or technological advancements. Despite these limitations, the Lorenz curve remains a valuable tool for understanding income inequality and informing policy decisions.
Read more
Assumptions and requirements of the Atkinson index
The Atkinson index is a measure used to evaluate income inequality, making assumptions that individuals' utility depends solely on their income levels. It relies on three key requirements: welfare dominance, symmetry, and the Pigou-Dalton principle. Welfare dominance asserts that a redistribution policy is desirable if it increases the welfare of individuals with lower incomes. Symmetry means that the index treats income reductions and increases equally. Lastly, the Pigou-Dalton principle states that income transfers from richer to poorer individuals should decrease inequality. While the Atkinson index offers valuable insights into income inequality, it's important to acknowledge its assumptions and requirements to properly interpret and apply its results.
Read more
Assumptions and Interpretations of Gini Coefficient
The Gini coefficient is a measure of income inequality that helps policymakers understand the distribution of wealth within a population. Assumptions and interpretations of the Gini coefficient are crucial for making informed decisions. One assumption is that inequality is a relevant concern, as the coefficient reflects disparities in income. Another assumption is that the Gini coefficient accurately captures the degree of inequality, as it considers the entire income distribution. Interpretations of the coefficient vary, with some arguing that a higher coefficient indicates greater inequality, while others believe it represents economic efficiency. Understanding these assumptions and interpretations is vital for policymakers aiming to address income inequality and promote a more equitable society.
Read more
