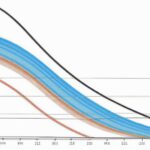
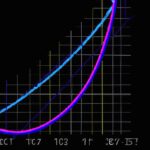
When starting a calculation, first gather all necessary information. Next, identify the required steps. Then proceed to perform the calculations accurately. Verify the results for correctness. If needed, double-check calculations. Ensure to use a reliable method. Regular practice improves calculation accuracy. Remember to stay focused and avoid distractions. Following these steps will lead to effective and precise calculations.
Table of Contents
(Mastering Formula Method for Nursing School & the NCLEX: Intro Guide)
When calculating mathematical problems, a systematic approach is essential. Begin by identifying the problem and its variables. Next, choose a suitable method or formula for computation. Carefully input the data and calculate step by step. Verify your results to ensure accuracy. Standard steps include addition, subtraction, multiplication, and division. Utilize parentheses to indicate the order of operations. Decimal points and fractions require special attention. Rounding off numbers may be necessary depending on the context. Complex calculations may require multiple steps or specialized techniques. Interpreting the results is crucial for understanding the implications. Practice and repetition enhance calculation proficiency. Understanding the underlying principles aids in solving diverse problems. Continuous learning and application refine calculation skills over time. Embrace challenges as opportunities for growth and skill development. Seek clarity and guidance when faced with difficult calculations. With patience and practice, mastery of calculation methodology is achievable. Celebrate successes and learn from mistakes along the way. Engaging with calculations fosters critical thinking and problem-solving skills. Embrace the journey of learning and expanding your mathematical abilities.
Calculation concepts
When delving into the realm of calculation concepts, we find ourselves embarking on a journey through the intricate pathways of numbers and logic. Calculations are not mere arithmetic exercises but rather complex puzzles begging to be solved, each holding its unique set of rules and principles.
At the heart of calculation lies understanding fundamental operations such as addition, subtraction, multiplication, and division. These pillars support more advanced mathematical processes like algebraic equations or geometric formulas. Imagine these operations as tools in a craftsman’s workshop – each serving a specific purpose, yet interconnected in their application.
Consider addition; it is the art of combining quantities to form a larger whole. Whether tallying up grocery expenses or summing up test scores, addition brings elements together harmoniously. Subtracting is akin to carving out pieces from a whole – it’s about discerning what remains after removal. Subtraction teaches us precision and elimination.
Multiplication amplifies our numerical universe by creating replicas or groups within sets. It expands possibilities exponentially with every repeated unit added—a dynamic force driving growth in mathematics.
Division acts as both a sword and shield—it divides wholes into parts while also protecting fairness among shares allocated equitably based on need or proportionality.
Beyond these basic operations lie deeper realms awaiting exploration: exponents that elevate numbers to new heights; logarithms that unravel exponential mysteries; calculus which deciphers rates of change at infinitesimal levels—each concept adding layers of complexity and beauty to calculations’ tapestry.
As we navigate this landscape filled with digits dancing like stars in an infinite sky, emotions may arise—anxiety when faced with daunting problems; joy when unlocking solutions hidden beneath layers of abstraction; satisfaction upon mastering once insurmountable challenges.
In conclusion, calculation concepts transcend mere computation—they sculpt logical reasoning skills honed through practice and perseverance. So next time you pick up your pencil or tap on your calculator, remember you’re not just crunching numbers but engaging in an ancient dance between mind and mathematics—a symphony where precision meets creativity in perfect harmony!
Error analysis
Error analysis is a crucial aspect of any calculation process. It’s like peering into the soul of your math work, revealing its flaws and imperfections. Picture this: you’re diligently crunching numbers, thinking you’ve nailed it, only to be blindsided by that pesky margin of error.
The first step in error analysis involves identifying the sources of potential slip-ups. Was it a rounding mistake? Maybe a misplaced decimal point or an overlooked negative sign? These tiny blunders can snowball into significant errors if left unchecked.
As you delve deeper into the labyrinth of calculations, uncertainty creeps in like mist on a chill morning. You start second-guessing yourself – doubting your arithmetic prowess. But fear not! Error analysis isn’t about pointing fingers; it’s about learning from mistakes and honing your skills.
Imagine watching a skilled tightrope walker gracefully crossing a chasm while balancing precariously above the abyss below. That’s what error analysis feels like – navigating through mathematical pitfalls with grace and precision.
Once you’ve pinpointed where things went awry, it’s time to rectify those missteps. Like a detective solving a perplexing case, you meticulously trace back your calculation path, searching for clues to unravel the mystery of errors past.
With each correction made, there’s a sense of satisfaction that comes from untangling the web of miscalculations. It’s akin to polishing a rough gem until it gleams with newfound clarity and brilliance.
Error analysis isn’t just about fixing mistakes; it’s also about fortifying your understanding of mathematical concepts. Through this process, you unearth hidden insights and deepen your knowledge base – transforming setbacks into stepping stones towards mastery.
Embrace error analysis as a friend rather than foe – an ally in your quest for numerical proficiency. Celebrate each corrected misstep as a triumph over adversity and watch as your confidence soars amidst the tangled webs of calculations.
Practical examples.
Alright, let’s dive into practical examples within the realm of calculation steps and methodology. Imagine you’re a chef following a recipe step by step to create a mouth-watering dish. That’s essentially what calculations are like – precise instructions to guide you from start to finish.
Let’s consider an everyday scenario where we need to calculate the total cost of groceries in our shopping cart. First, jot down all items on your list with their respective prices per unit – tomatoes at $2 each, bread for $3, and milk priced at $1 per liter. Now comes the fun part: multiplication! Multiply the quantity of each item by its price per unit; say 5 tomatoes x $2 = $10.
Next up is addition – simply sum up all these individual costs together (tomatoes + bread + milk) to get your final grocery bill. Voilà! You’ve successfully applied basic calculation methodology in real life without breaking a sweat.
Now, let’s shift gears and delve into a more complex example involving calculating compound interest on investments. Picture yourself as an aspiring investor keen on growing your savings exponentially over time through smart financial decisions.
To calculate compound interest, you’ll need three key inputs: principal amount invested ($1000), annual interest rate (5%), and investment period (3 years). The magic formula involves multiplying the principal amount by one plus the annual interest rate raised to the power of the number of compounding periods (in this case quarterly).
As you crunch those numbers diligently, anticipation builds within you as you discover how your money can work for you effortlessly through compounded growth. Each calculation brings clarity and excitement about potentially reaching new financial milestones sooner than expected.
In both these scenarios, whether it’s tallying grocery expenses or projecting investment returns, calculations serve as powerful tools that empower us in decision-making processes while unveiling hidden insights behind seemingly mundane numbers.
So next time you find yourself faced with numerical challenges in daily life or professional settings, remember that mastering calculation steps and methodologies not only simplifies tasks but also opens doors to boundless possibilities yet unexplored.
(Standard Method Dwelling Unit Calculations Part 1)
Tools and techniques
When diving into the realm of calculations, having the right tools and techniques at your disposal can make all the difference. Imagine yourself equipped with a trusty calculator, its buttons worn from countless calculations, yet it still faithfully helps you navigate through numerical complexities.
As you embark on these mathematical journeys, pen in hand (or fingers poised over a keyboard), ready to decipher equations that may initially seem like hieroglyphics. Each step is a puzzle waiting to be solved – an intricate dance between numbers and symbols.
You might find solace in spreadsheets, where columns neatly align numbers like obedient soldiers awaiting orders. Formulas are your weapons here; each keystroke imbued with purpose as you command cells to yield their secrets through calculated precision.
Graph paper becomes your canvas when dealing with functions and plotting points – transforming abstract concepts into visual representations that breathe life into dry mathematical theories. Lines intersecting, curves flowing gracefully across x and y axes like dancers twirling in perfect harmony.
In this world of calculations, your mind is both sword and shield – slicing through complex problems with sharp logic while guarding against errors lurking in the shadows of careless mistakes. Precision becomes paramount as even a misplaced decimal point could lead to cascading inaccuracies.
With each calculation comes a sense of accomplishment or frustration – depending on whether your journey ends in triumph or sends you back to the drawing board for recalibration. But through it all, there’s an undeniable thrill in unraveling mathematical mysteries one step at a time.
So next time you embark on a calculation quest armed with tools and techniques galore, remember that behind every number lies a story waiting to be told – an equation eager to reveal its secrets if only you’re willing to listen closely enough.
Variables and constants
When delving into the realm of calculations, understanding variables and constants is key. Imagine them as characters in a mathematical story – one dynamic and ever-changing, the other steadfast and unwavering.
Variables are like chameleons; they shift and adapt depending on the situation. They are placeholders for unknown values waiting to be unveiled through the magic of computation. In an equation, variables give us flexibility, letting us solve problems across a spectrum of scenarios with finesse.
On the flip side, constants are the bedrock of stability in this chaotic math universe. They hold their ground, maintaining a fixed value throughout our calculations. Think of them as anchors keeping our equations grounded amidst the swirling sea of numbers.
In any calculation journey, we encounter these two essential elements hand-in-hand, guiding us through twists and turns towards solutions. Variables invite speculation while constants provide certainty – together creating harmony within mathematical chaos.
As we navigate through complex formulas or intricate algorithms, variables tempt us with their mysterious allure while constants offer assurance in turbulent times. The dance between them forms the essence of problem-solving – a delicate balance that requires both intuition and logic to master.
Picture solving an equation as exploring uncharted territory where variables lead you down unforeseen paths while constants act as your faithful companions steering you back on course when needed.
Emotions run high in this numerical adventure; frustration when variables evade easy solutions yet elation when constants bring clarity to once murky waters. It’s a rollercoaster ride through peaks of triumphs and valleys of challenges that ultimately culminates in a sense of accomplishment when equilibrium is found amongst these mathematical comrades.
So next time you embark on a calculation quest, remember the interplay between variables and constants – embrace their duality, harness their power, and let them guide you towards unlocking the secrets hidden within numbers’ enigmatic realm.